Games On / Standing Room Only - Teacher Notes
Games On
The organizers of the 2012 London Olympics need your help!
They want all the athletes to be present at the opening ceremony, in the centre of the athletics track.
Can everyone fit in?
Explain why or why not, in a letter.
At the 2004 Olympic Games in Athens 11,000 people from 296 countries took part. Assume the same number take part in 2012.
The stadium has the dimensions shown on the diagram below [square sides are 10 metres].
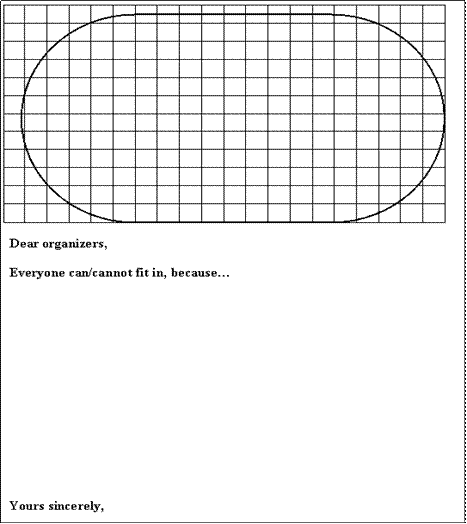

Standing Room Only
Ron claims that the whole population of China could stand on the Isle of Wight.
The CIA World-FactBook web page gives the population of China to be : 1,321,851,888 (July 2007 estimated.)
And Wikipedia.org gives the area of the Isle of Wight as: 380 kmē (146.7 sq mi)
Is Ron's claim plausible?

Teachers' notes for Games On and Standing Room Only
THROUGHOUT - AVOID SPURIOUS ACCURACY!
Games On [about 40-60 mins]
Using the Task
Class Organisation
We recommend that pupils work in groups of 3 or 4.
Lesson Activities
A: Exploring the problem - generate discussions about lots of unknowns, for example:
- how long will the athletes have to stand for?
- Can all the space can be used? - do we need space corridors to allow people to get out in an emergency?
- Do we need space for displays in the ceremony?
The video of the opening ceremony in Barcelona gives the pupils some visual stimulus which may help ground the discussions - bear in mind that in mathematics, pupils are normally given information explicitly - here, there is quite a lot of information contained contextually in the video. [10-15 minutes]
B: Elicit ideas on the key things to find out [how many people in a unit space, and how many unit spaces are available]. [10-15 minutes]
C: Practical activity - people in a unit space
Use a corner of the classroom and a desk to create a 3-sided enclosure which is roughly 1m by 1m. Ask pupils how many people could stand in it; get volunteers to stand in the space. This activity works as a whole class demonstration, or as part of group work.
Encourage pupils to:
- decide on a range which they think is reasonable for adult athletes [something like 3 to 5]
start off by working on the simplest version of the problem - assume that all the space can be used. [10-15 minutes]
D: Group work/individual activity
If pupils finish early, they should be encouraged to check their work, and to ensure that the letter to the organisers is well crafted (elicit ideas on the key things the organisers will want to know; clarity of explanation is important).
How much space is available?
- pupils have squared paper, so counting squares is one possibility.
- Pupils might approximate the shape to a rectangle.
- they only need a rough idea of area, so 'calculating circle areas' is fine, but probably not necessary. If students DO calculate the area of a circle, you might suggest they use 3 as an approximation for pi.
- Manipulating areas on an IWB may be a help here in encouraging pupils to make appropriate approximations. [answer: roughly 160 m by 100 m].
Rough estimates give about 16,000 square metres for 11,000 people, so there is plenty of room.
The number of people in the square metre isn't crucial - everyone has more than a square metre.
The calculation of the area need not be accurate to answer this question (within 25% is probably good enough) given the large uncertainty in the estimate of the number of people in a square - pupils could underestimate the area by a factor of 2 or more, and still come to the same conclusion.
What if pupils want to leave space for displays and emergency exits? There is easily enough room, so there is no need to do any calculations (of course, pupils who disagree can be encouraged to do the sums!).
[10-15 minutes]
Class Review and Discussion
During the group work, make a mental note of the different methods being used. Ask for descriptions and answers that demonstrate the differences. Ask each group for their estimates and their answers.
You might ask some pupils to read out their letters to the Organizers
Key ideas
- Breaking the task up into components
- Different strategies for the approximation [rectangles versus careful calculations involving circles]
- The link between appropriate accuracy of measurement and the clarity of the answer. If we assume that all the space can be used, it is clear that everyone can fit. When the intangibles in the task are explored (such as not using all the space e.g. for safety corridors) the answer is the same. If the first answer was a close call, then all the approximations used would have to be looked at very carefully
- If different groups use different methods and get the same answer, this is good evidence that the answer is correct!
- If 5 people can stand in a 1 metre square, then 5 x 100 x 100 can stand in a 100 metre square (generally, if you change the length of each side by some factor, then the area goes up as the square of the factor).
Discuss the critical things to include in the letter to the organizers - exactly what will the organisers want to know? assumptions made (are they plausible), calculations, the answer, how confident they are about their answer. The 'confidence' issue hangs on the sensitivity of the conclusion to the estimates they have made.
More Information
http://www.athensinfoguide.com/olympicfacts.htm
Mathematical Links
Scaling
History of mathematics: calculating the area of a circle via bounded estimates based on internal and external polygons - 'squaring the circle'.
Standing Room Only [about 30 mins]
This task can be used as an extension task for pupils who finish early, or as a task in its own right as a follow on (otherwise it will need longer than 30 minutes)
Key skills
- working with different units
- trying different approaches, and seeing if they produce the same conclusions
On spurious accuracy: get pupils to discuss the likely accuracy of the estimate given for the population of China.
On Units: the Isle of Wight is in km2 (or worse, square miles!), other work has been in square metres.
On Methods to find the number of persons:
- Some will look at building up a series of square metres with people, in rows, then moving to the next row, and working out what will happen on the map. For example, if students decide that 4 people can stand in a square metre, they might construct a table like this one.
| 4 | 4 | 4 | 4 | 4 | .. | .. | .. |
| 4 | 4 | 4 | .. | ||||
- A second approach is to calculate the length of the side of the square, given the area of the IoW in square kilometres, then convert to metres. Each square metre can hold 2 people on each side, so the total number of people can be calculated.
- A third approach is to calculate the number of people on each side of a 'people square'. Each side needs to have SQRT (population) on it. Then one calculates how many metres are needed on each side (at 2 per metre). This can then be compared to the available land.
Pupils may want to correct for rivers, steep slopes etc.
http://en.wikipedia.org/wiki/Isle_of_Wight gives the area as 380 km2
Does the evidence support the first statement?
There is more to over-population than standing room. Some of the issues which pupils generate could be used as seeds for adventurous Plausible Estimation tasks. E.g. Energy, housing, water, food, education, pollution.
Key Ideas to Discuss
Conversion of units.
Different solution methods - and their evaluation.
The spurious accuracy of an estimate in billions of people, given in the CIA website.